Instrument Simulation Laboratory
Tank Level Measure simulator based on a Pressure Transmitter
You can share this lab through:
1.Tank Level Measure simulator
The objective of this laboratory is to have a tool to understand the relationship between a measurement located in the field and its visualization in the control room. Many times it is necessary to make a change to an instrument or to a range related to the instrument, either in the field or in the control system. This laboratory allows us to experiment and see what the impact of our changes would be in the different related measures, but without affecting our process.
How many times have we wanted to test what would be the change in our control system if we made a change in a variable in the process?
2.How does this simulator work?
Our laboratory allows us to simulate the evolution of the level measurement of a tank (R101) with respect to time. The R101 tank has an effective height of 10 meters. To measure the level we have a relative pressure transmitter that allows us to obtain the hydrostatic pressure of the tank.

This pressure sensor uses a metallic membrane sensitive to pressures between 0 and 10,000 mmwg. Our pressure measurement is sent to the DCS through a current loop signal between 4 and 20 mA. In the control system, the measure is represented as a percentage from 0 to 100%.
2.1.Start and Stop Simulation
We can start and stop the simulation through the Start and Stop buttons.

To completely reset the content of the graphics it is necessary to reload the page.
2.2.What information do the graphs show?
The first graph shows the real level of the tank, in green we will see the evolution over time of the process variable, our level. In this case our level measurement in the field is carried out through pressure measurement, applying the principle of hydrostatic pressure measurement we can determine through of pressure our measure of level.
The second graph shows us the evolution of the intensity managed by the transmitter with respect to time, typically on a scale of 4 to 20 milliamps.
The third graph shows us the relationship between the process measurement observed in the control room, expressed as analog input to the DCS versus the time.
SIMULATION TIME [s] : 00:00 - STOPPED
Field Pressure Measure
Current Loop Signal
DCS Level Measure
2.3.What additional parameters do we have?
For each of the graphs it is possible to define the measurement range, the range of the displayed measurement depends on the type of variable, for example if we are measuring the pressure expressed in millimeters of water column for a range of 10 m real, our equivalent pressure it will be from 0 to 1000 mmwg.
Thus, each of the measurements and each of the graphs will have its calculation range. The display range is equal to the calculation range plus 10%.
At the bottom we show you three examples of the use of this laboratory, we hope you enjoy this laboratory.
2.4.Basic Handling
For each graph there is a cell called NF. NF is the acronym for Noise Factor, the value of NF can range from zero to infinity. A value of NF equal to zero implies eliminating the noise from the signal.

In addition to this cell we have a read-only cell in which the current value of the signal related to the graph appears, so for the first graph we will have a cell called Out with the last value of the related graph.

In order to define the initial value of the R 101 tank, we have a cell called Current Measure that allows defining the tank pressure measurement (in mmwc). For example, if we want to define the tank level at 4 m, we must enter its equivalent in mmwc which is 4000.

3.Graphical interpretation of signal ranges
A helpful illustration for students in understanding analog signal ranges is to consider the signal range to be expressed as a length on a number line. For example, the common 4-20 mA analog current signal range would appear as such:

If one were to ask the percentage corresponding to a 14.4 mA signal on a 4-20 mA range, it would be as simple as determining the length of a line segment stretching from the 4 mA mark to the 14.4 mA mark:
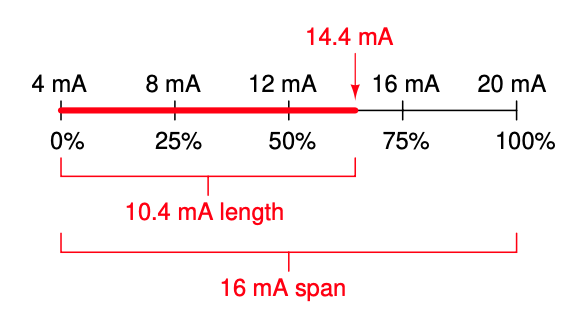
As a percentage, this thick line is 10.4 mA long (the distance between 14.4 mA and 4 mA) over a total (possible) length of 16 mA (the total span between 20 mA and 4 mA). Thus:

- Rating: 4.7 - 50 reviews
5. References:
- MEIER,F.A. (2004) Instrumentation and Control Systems Documentation
- SAMSON (2013) Terminology and Symbols in Control Engineering
- KUPHALDT, TONY R. (2019) Lessons In Industrial Instrumentation
- BATTIKHA,N. E. (2006) Condensed Handbook of Measurement and Control
- DOUGLAS,O.J. (2005) Applied Technology and Instrumentation for Process Control
- DUNN,W.C. (2006) Introduction to Instrumentation Sensors And Process Control
- GOETTSCHE,L.D.(2005) Maintenance of Instruments and Systems
- ANSI/ISA-5.1-1984, Instrument Symbols and Identification, ISA, Research Triangle Park, NC, 1984.
- HUGHES,T. (2002) Measurement and Control Basics
- ISA (2012) Successful Instrumentation and Control Systems Design
- LOVE,J. (2007) Process Automation Handbook A Guide to Theory and Practice
- SIEMENS (2012) Procidia Control Solutions SAMA Diagrams for Boiler Controls
Another articles that may interest you:
- A complete list of the indispensable books that every professional in process control engineering must have, with prices and direct links to buy them if you want.
- In Flow Rate Calculator you can calculate the volumetric flow rate of any liquid or gas through a specific pipe diameter and download results.
- Volumetric Flow Converter, another of our useful free online calculators .
- If you need a Restriction Orifice Calculator, these are your tools.
- How to convert actual flow to normal flow?, How to convert standard cubic meter to normal cubic meter?, How actual to normal flow conversion works? If you want to answer these questions don't forget to read Difference between Actual, Standard and Normal Flows
- In Introduction to Hazop Metholodogy, there is all the information about Hazop metholodogy that ever instrumentation technician should know.